Right-hand rule - a rule which is used for defining direction or “handedness” of magnetic field or magnetic force with relation to the direction of conventional electric current or movement of positive electric charges.^1)2)3)4)5)6) For negative charges such as electrons, the direction of the magnetic force is reversed.⁷⁾⁸⁾

Orientation of magnetic field (H or B) with respect to the current I follows the right-hand rule

^{S. Zurek, E-Magnetica.pl, CC-BY-4.0}

There are two main versions of the “hand rule”: one for direction of magnetic field, and another one for direction of magnetic force. They are typically represented as two separate rules, but are ultimately resulting from the same underlying principle.

There are many different depictions of these rules in the literature, by using: curled fingers, outstretched fingers, open palm, etc., with several mnemonics taught to pupils and students to help remembering what directions should be assigned to a given finger, thumb, or palm. Also, typically the direction of magnetic force in the motor and generator is shown with left or right hand. Consequently, there are many names for these rules: Fleming's right-hand rule, Fleming's left-hand rule, Ampere's right-hand grip rule, thumb rule, corkscrew rule, end rule, clock rule or slap rule.⁹⁾

All these rules are equivalent, because the direction of the physical magnetic force (Lorentz force) is always the same.

The “hand rules” for directions of magnetic force were proposed in 1890 by John Ambrose Fleming.¹⁰⁾¹¹⁾

Definition of left-handed and right-handed system of coordinates, with the vector cross product $\vec{c}=\vec{a} × \vec{b}$ expressed with fingers outstretched at right angles

^{S. Zurek, E-Magnetica.pl, CC-BY-4.0}

At a fundamental level it is not possible to calculate, in an absolute way, a value for binary quantities such as positive/negative (electric charge), clockwise/anticlockwise (direction of rotation), up/down (side of a surface), etc. They can only be defined with relation to each other, or to some closely related direction in the same system of coordinates.

For a three-dimensional system of coordinates also the orthogonality of the three axes can be defined as being “left-handed” or “right-handed”. It has been generally accepted (by convention) that unless stated otherwise the right-hand system is assumed for some calculations published in the literature. This is because certain mathematical functions (such as the vector cross product) would return a negative value if the calculation was made in the opposite system.^12)13)14)

A right-handed system can be defined with two fingers and a thumb, placed at right angles to each other:

• the index finger points in the positive direction as the first axis (x)
• the middle finger points in the positive direction of the second axis (y)
• the thumb points in the positive direction of the third axis (z)

Positive direction for circulation of a vector around the path C is also defined with the right hand

^{S. Zurek, E-Magnetica.pl, CC-BY-4.0}

The vector cross product of two vectors $\vec{a}$ and $\vec{b}$ is a vector $\vec{c}$ pointing in the third orthogonal direction, so the same right-hand rule can be used to distinguish the direction of the resulting vector.¹⁵⁾¹⁶⁾

“Circulation” of a vector in the right-handed system is also defined by the right-hand rule. The positive direction of the unit vector $\hat{n}$ normal to the surface $a$ is as indicated by the thumb, and the curled fingers indicate in which direction the integral should be taken around the path $C$.¹⁷⁾¹⁸⁾

Calculations of magnetic forces in three-dimensional space involve vector calculus, which by convention operates in a right-handed system, therefore right-hand rules (as outlined above) should be used accordingly.

Circulation of magnetic field vector with respect to the current $I$ follows the right-hand rule, which is also applicable if the vectors change places: magnetic field circulates around a straight wire with current, or magnetic field is generated along the axis of a current loop or a solenoid

^{S. Zurek, E-Magnetica.pl, CC-BY-4.0}

For the direction of magnetic field the rule is similar to that of circulation of a vector.

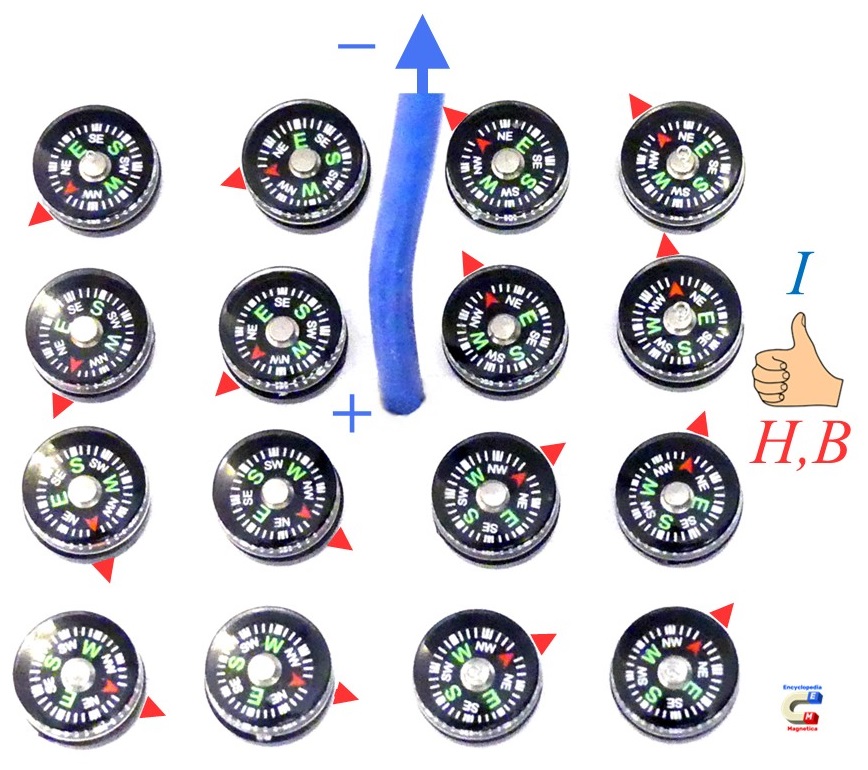
The right hand is depicted with the thumb following the direction of the current in a straight wire, and curled fingers show the direction in which the magnetic field (flux density B or magnetic field strength H) circulating around the wire.¹⁹⁾

The rule is equally applicable to the alternative situation in which the current flows in a loop (or a solenoid) and the magnetic field is generated along the direction of the axis of such loop.²⁰⁾²¹⁾

The rule then even applies if the thumb points in the direction of the field, and the curled fingers show the direction of current in the loop.

For such loop, the magnetic poles N and S appear at each end, and they can be distinguished by the stylised letters with arrows at their ends, which show the apparent direction of “rotation” of current in the loop. As shown in the illustration, when looking from the end marked with “N”, the current appears to flow in the anticlockwise direction. At the same time, when looking from the end marked with “S”, the current appears to flow clockwise.

Some illustrations show the hand gripping the solenoid or wire, hence the origin of the name grip rule.

The thumb points in the same direction as magnetic north pole when applying the right-hand rule to a solenoid; the magnetic poles are denoted by N and S

^{S. Zurek, E-Magnetica.pl, CC-BY-4.0}

Magnetic poles N and S are created for any loop of electric current

^{S. Zurek, E-Magnetica.pl, CC-BY-4.0}

Compass needles follow the direction of magnetic field (B or H) around the wire with current I

^{S. Zurek, E-Magnetica.pl, CC-BY-4.0}

The other application of the right-hand rule is for the analogy of the direction of magnetic force developed on a moving charged particle or a wire with current placed in magnetic field. In some literature this rule is discussed as if it was a separate rule from the one described in the section above, but it results from the same principles.

Lorentz force F acting on an electric charge q moving with velocity v is due to the electric field E and magnetic field B (magnetic flux density):

In the absence of electric field (E=0) or if its effect is negligible, the equation reduces to the one below, and the field which satisfies the action of the B vector is assumed to be the definition of magnetic field:^22)23)24)

This equation involves the vector cross product which is calculated in a right-handed system of coordinates and therefore the magnetic force follows the same right-hand principle as for the definition of the right-handed system itself - by using two fingers and a thumb placed at right angles to each other.

The index finger shows the direction of the first vector, which in this case is the direction of the original movement of the positive charge $q$ which constitutes conventional electric current $I$. In an ordinary conductor if some voltage is applied across it the electrons will flow in the opposite direction, but it is the conventional current (flowing from plus to minus) which is taken into account here.

The middle finger shows the direction of the second vector, which is the direction of magnetic field $B$.

The thumb points in the third orthogonal direction, namely in the direction of the magnetic force $F$ acting on the charge moving in magnetic field.

If the electric charge has a negative value (e.g. electron) then the force acts in the opposite direction.

If both the direction of movement, and the polarity of charge is reversed, then the force acts in the same direction. For example, the illustration on the right shows the situation for a hypothetical positive charge moving from plus to minus due to the current in the wire, and the force acts upwards. In the same wire, the electrons would flow from minus to plus, in the opposite direction to the conventional current. And because two of the variables were changed (polarity of charge and its direction of movement) then the force will still act upwards on such electrons. In fact, in a real wire only the negatively charged electrons move, as the positively charged protons remain bound to the atoms, which are stationary with respect to the body of the wire.

It should be noted here that the magnetic force for a positive charge always follows this rule, regardless of any other conditions. Therefore, exactly the same right-hand rule is applicable to both motors and generators.

In motors, the conventional movement of charges is caused by the conventional current which flows along the conductor in question. The magnetic force will push the conductor in the third orthogonal direction, causing physical movement of the conductor and generation of useful output torque.

However, in generators, the charges are originally moved because the wire is pushed by some input torque. The charges move together with the wire, and the magnetic force pushes them along the wire, thus creating an electromotive force (EMF).

Therefore, the right-hand rule is easy to remember, because the index finger always show the original direction of the charge movement (as if the index finger was pushing the charge), the middle finger always shows the direction of magnetic field, and the thumb always shows the direction of magnetic force. This logic is consistent with the application of the vector cross product, as explained above for the right-handed system of coordinates.

The original “hand rule” introduced by Fleming defined the direction of the induced electromotive force. In the book “Magnets and electric currents” published in 1902, Fleming gave the following description and mnemonics linking letters to names of fingers and variables. In this publication only the right-hand rule was defined, as shown in the illustration.²⁶⁾

However, nowadays there are publications which refer to the Fleming's left-hand rule for magnetic force in motors and right-hand rule for generators.^27)28)29)

One version with the left hand is sometimes colloquially referred to with the mnemonic “FBI”, which makes it easier to remember because in this particular version:³⁰⁾³¹⁾

• Motion in the direction of the thuMb (for force F)
• Flux or Field acts in the direction of the First finger (for magnetic field B)
• Current in the direction of the seCond finger (for current I)

So progressing in the sequence thumb-first-second the mnemonic F-B-I is created.

The name corkscrew rule compares the directions to the rotation of corkscrew or right-handed screw

^{S. Zurek, E-Magnetica.pl, CC-BY-4.0}

However, it should be emphasised that all these different depictions are correct only with respect to the directions and numerical values. Simplified scalar equations such as: $F = B·l·I$ and $EMF = B·l·v$ work only under the implicit assumption that all the values are represented by orthogonal vectors, which is not true for a general case (if the charge moves along the magnetic field then there is no magnetic force). Hence, additional information such as cosine of the angle between the quantities has to be included.³²⁾ Whereas with vector notation, the right-hand rule is effectively illustrating the vector cross-product which is true under any conditions, if the vector multiplication is taken into account correctly.

Sometimes, the right-hand rule is also compared to the direction of rotation which is made when driving in a corkscrew or a right-handed screw (i.e. any ordinary screw or thread such as a lid on a jar or bottle) to tighten it, or as if a drilling action was taking place in the clockwise direction (looking from above).³³⁾

The same rule represented by left or right hand requires different fingers to be assigned to different variables

^{S. Zurek, E-Magnetica.pl, CC-BY-4.0}

This multiplicity creates unnecessary complication and confusion, which makes more difficult understanding of the whole subject.

This is most likely because the same kind of orthogonality can be represented by various configurations of fingers, on either hand. Fingers can be outstretched orthogonally, or the palm can be flat (hence slap rule).

As explained above, all these different versions (right or left hand, open palm or outstretched fingers) are exactly equivalent as far as the directions are concerned, because magnetic force always acts in the same way.^34)35)36)37)

• Magnetic force