Table of Contents
Solenoid
| Stan Zurek, Solenoid, Encyclopedia Magnetica, https://e-magnetica.pl/doku.php/solenoid |
| reviewed by Branko Koprivica, 2022-03-01 |
Solenoid - a coil typically shaped as a round cylinder,1)2) but also of a rectangular cross-section.3)4)
There are two main meanings of the name “solenoid” which can be encountered in the literature: as the source of magnetic field (without any magnetic materials), and as the electromagnetic actuator (typically with ferromagnetic components).
Solenoid coils are used as a source of uniform magnetic field in research. The value of magnetic field at the centre of a solenoid can be calculated from analytical equations, expressing them as a function of geometric dimensions and electric current in the coil. Thus, a known value of field can be generated, precise in the sense of absolute accuracy, so that it can be used for calibration of other sensors.5)6)
Solenoid actuators are used to provide a mechanical force, typically along a straight line, but also along an arc. Similarly to electronic relays, solenoid actuators are typically designed to operate from standardised DC voltages such as 12 or 24 V, providing defined force over certain distance.7)8)
The magnetic flux density B is said to be “solenoidal field” because the imagined magnetic field lines form closed loops, without beginnings or ends. The field is “sourceless” because for all points in space the divergence of the vector field B is zero.9)10)11) This is different from the electric field E which has specific sources: the positive and negative electric charges.
| → → → Helpful page? Support us! → → → | PayPal | ← ← ← Help us with just $0.10 per month? Come on…  ← ← ← |
Loop of current
A current loop is one of the basic structures which can be used for generation of known magnetic field. The intensity of the field at the centre of the loop on its axis reaches a maximum, but the spatial variability is typically too great to be useful for experiments which require uniform field.
The “infinitely thin” loops are sometimes referred to as “filamentary coils”.12)13)
However, the useful volume can be increased by arranging multiple loops in a tubular configuration.
S. Zurek, E-Magnetica.pl, CC-BY-4.0
Helmholtz and Maxwell coils
S. Zurek, E-Magnetica.pl, CC-BY-4.0

S. Zurek, E-Magnetica.pl, CC-BY-4.0
A Helmholtz coil is substantially a pair of current loops, arranged such that they are positioned in parallel to each other, on the same axis, and separated exactly by a distance equal to the radius of the loop.
In practice, the coils are wound with multiple turns so that lower current can be used from a power supply. However, both coils have to have the same number of ampere-turns (same current, same number of turns).
Such arrangement provides a significant volume of uniform field. Additionally, pairs of coils can be arranged in an orthogonal manner so that the field can be generated in any direction in 3D, which can be used for example for compensating out the Earth's magnetic field.14)
Helmholtz coils are widely used in research, because of the large volume of uniform field, the openness of the structure (easy access) and low cost.15) However, the intensity of obtainable field is limited, as compared to solenoids.
Further improvement of uniformity of field over larger volume can be achieved by adding more loops of current, in an appropriate arrangement, distributed over a spherical shape. The Helmholtz pair is effectively also distributed on a sphere, but with the minimum number of loops (just two). At the other extreme is a full sphere, but it is not used in practice due to complexity of making of such coil and the access to its inside.16)
Three such co-axial coils are know as a Maxwell coil. They have to be arranged such that the largest coil in the middle has radius R and the two smaller ones have radius $r = R · \sqrt{4/7}$ and the distance between the coils should be $d = R · \sqrt{3/7}$. The current can be equal in each coil, but the ratio of ampere-turns must be matched so that outer/inner = 49/64 (if the current is the same the turns must have the same ratio of 49/64).17)18)
With four coils, also know as a double Helmholtz coil, they can be also distributed over a sphere, further increasing the uniformity.19)
Cylinder of current
Uniform magnetic field of analytically calculable value can be generated by a coil which is wound on a circular cylinder. Such cylindrical solenoid can be used as a precise source of magnetic field, suitable for calibrating other magnetic sensors.20)
Such a “cylinder of current” or “tube of current” is a similar approach in calculation of magnetic field to the “sheet of current” for a flat structure. This is different than the “spherical coil” approach mentioned above, but it also can be thought of as a series of current loops arranged co-axially. Both Biot-Savart law or Ampere law can be used for calculation of the magnetic field in a solenoid.21)22) The Biot-Savart law can be used to calculate values of magnetic field at different positions rather than just along the axis of symmetry.23)
Typically, a solenoid is wound on a tube which is empty inside, so that other devices or structures can be inserted. Therefore, also the inside of such solenoid is non-magnetic, with permeability of the air (or any other gas or vacuum used for the experiments as the surrounding medium), which for most engineering applications can be assumed to be equal to unity with a negligible error.24)
Under such conditions, the magnetic field inside the solenoid is directed along the axis of the cylinder. The field is uniform and the value at the geometric centre of the solenoid can be calculated from the approximate formula:
| Magnetic field strength H inside an ideal solenoid with number of turns N and length L |
|
|---|---|
| $$H(t) \approx \frac{N·I(t)}{L} $$ | (A/m) |
| where: $H(t)$ - instantaneous values of magnetic field strength $H$ (A/m) vs. time $t$ (s), $N$ - number of turns of the solenoid (unitless), $I(t)$ - instantaneous values of electric current (A) flowing through the solenoid wire, $L$ - length of the solenoid (m); the equation is valid only if $L \gg d$, and $d$ - diameter of the coil (m) | |
For solenoids of finite length the field at the centre is lower the shorter is the solenoid, so appropriate corrections have to be made as discussed below in detail.
There is a direct proportionality between the value of electric current flowing on the solenoid, and the value of generated magnetic field. For a solenoid in a non-magnetic medium the relationship can be expressed with a single proportionality constant such that:25)
| Proportionality of magnetic field in a solenoid | |
|---|---|
| $$H(t) = k_H · I(t)$$ | (A/m) |
| $$B(t) = k_B · I(t)$$ | (T) |
| where: $H(t)$ - magnetic field strength $H$ (A/m) as a function of time $t$ (s), $k_H$ - proportionality constant (1/m), $I(t)$ - electric current $I$ (A) as a function of time $t$ (s), $k_B$ - proportionality constant (T/A) | |
For the calculations of an infinite length the equations are typically expressed with the variable of “number of turns per unit length” $n$ (turns/m), because otherwise the infinite “number of turns” $N$ could not be represented correctly. However, for a finite length of uniformly wound turns the two values are equivalent because $n = N/L$ (turns/m).
The magnetic field has a large amplitude inside the solenoid, and much lower outside. For an infinitely long solenoid the magnetic field outside approaches zero. Magnetic field at the centre of a solenoid has only the axial component (the radial component is zero).26)
Inductance of solenoids
Inductance is related to the concept of magnetic permeance, a reciprocal of magnetic reluctance. From a general viewpoint, self inductance can be calculated as the ratio of magnetic flux linkage and the current in a given coil.
However, for magnetic circuits of uniform cross-sectional area the calculations simplify considerably, and can be related to the magnetic path length, area, and magnetic permeability of the medium.
For a solenoid without ferromagnetic materials (e.g. in vacuum or air), or surrounded completely (inside and outside) by a uniform medium (including ferromagnetic) the inductance is proportional to the length of the solenoid.27)
However, if a gapped magnetic core is present, then most of the energy is stored in the magnetic field in the air gap and thus the length of the air gap dictates the value of inductance of such solenoid, winding, or coil.28)
| Inductance of solenoid in uniform medium | Inductance of solenoid with a gapped core | unit |
|---|---|---|
| $$L = \frac{μ_a · μ_0 · N^2 · A }{l}$$ | $$L \approx \frac{μ_0 · N^2 · A }{l_{gap}}$$ | (H) |
| where: $L$ - inductance (H), $μ_a$ - apparent magnetic permeability (unitless) equal to relative magnetic permeability for uniform medium, $μ_0$ - magnetic permeability of vacuum (H/m), $N$ - number of turns of the coil (unitless), $A$ - cross-sectional area of the coil or magnetic core (m2), $l$ - magnetic path length (m) equal to the length of the air-cored solenoid or length of the air gap $l_{gap}$ | ||
S. Zurek, E-Magnetica.pl, CC-BY-4.0

S. Zurek, E-Magnetica.pl, CC-BY-4.0
Mechanical forces
Magnetic force acting on conductors with electric current is such that the wires with parallel current attract each other, and the wires with anti-parallel current repel. Therefore, a solenoid energised with a DC or low-frequency current is compressed along its axis, but there is also a radial force acting outwards.
The mechanical forces are important for high-power transformers in which buckling of solenoid-like windings or conductors in them can lead to damaging high-voltage insulation and a catastrophic failure of the whole machine. 29)
At very high frequencies (approaching transmission line limit due to speed of light) there could be additional forces for reacting with the surrounding electromagnetic field, due to propagation delays.30)
Thin solenoid

S. Zurek, E-Magnetica.pl, CC-BY-4.0
If the wire (or conductor) diameter is much smaller that the diameter of the solenoid, then the solenoid is referred to as “thin”.31)
The ratio of the length of the solenoid and diameter still needs to be taken into account, but just one diameter can be used in the equation, which is applicable in most solenoids wound for example with a single layer of enamelled wire for laboratory purposes.
For an “infinitely long” solenoid, such that its length is much greater than its diameter $L \gg d$ the magnetic field strength at its centre tends to the “ideal” value of $H = \frac{N·I}{L}$.
However for real solenoids the real ratio $L/d$ has to be taken into account. For example a ratio L/d = 10 produces a relative field of 0.995 instead of the ideal 1.000 (difference of 0.5%).
The extent of the uniformity of magnetic field inside the solenoid depends on the L/d ratio, as illustrated in the graphs, calculated with the equations included below.
A the edges of the solenoid the field reduced to around 50%, and outside of the solenoid to much smaller values. At sufficiently large distance (much larger than the length of the solenoid) the field will behave as that of a magnetic dipole - reducing proportionally to the cube of distance.
Calculator of H along axis of round "thin" solenoid
| | Stan Zurek, Calculator of H along axis of round “thin” solenoid, Encyclopedia Magnetica, https://www.e-magnetica.pl/doku.php/calculator/solenoid_thin, {accessed: 2025-09-27} |
| Magnetic field strength H of a circular “thin” solenoid along its axis | ||
|---|---|---|
| Source: [1] Fausto Fiorillo, Measurement and Characterization of Magnetic Materials, Academic Press, 2005, ISBN 9780122572517 | ||
| [1], eq. (4.9), p. 110 | $$ H(t,x) = \frac{I(t) · N}{2·L}· \left( \frac{L + 2·x}{\sqrt{ d^2 + (L+2·x)^2}} + \frac{L - 2·x}{\sqrt{ d^2 + (L-2·x)^2}} \right) $$ | (A/m) |
| where: $I(t)$ - current (A) at time $t$ (s), $N$ - total number of turns (unitless), $L$ - length of the solenoid (m), $d \approx D$ - diameter of the solenoid (m), $x$ - location (m) from the centre of the solenoid (the centre is located at the point x = 0) | ||
Notes: This equation is valid only for uniformly wound solenoid, with infinitely thin wire. The instantaneous values of H are directly proportional to the instantaneous values of I. The value of B(μ0) is for vacuum.
| → → → Helpful page? Support us! → → → | PayPal | ← ← ← Help us with just $0.10 per month? Come on…  ← ← ← |
Thick solenoid

S. Zurek, E-Magnetica.pl, CC-BY-4.0

S. Zurek, E-Magnetica.pl, CC-BY-4.0
In a case where the thickness of the winding is not negligible, as for example it would be for a multi-layer coil, then the difference between the outer and the inner diameters is significant and thus it needs to be taken into account.32)
In a general case, all solenoids can be treated as “thick” because the wire or conductor used for making a solenoid has a finite thickness, which can be included in the calculations.
Generation of large magnetic fields requires use of very “thick” solenoids, because the power loss in the conductor of the solenoid becomes significant. Therefore, the design of the solenoid for very high fields becomes a compromise between the required magnetic field value, the heat dissipation in the conductor due to resistive heating, and the mechanical forces acting on the assembly.
The highest possible magnetic field can be obtained by an arrangement known as the Bitter electromagnet which is made from a series of split copper disks connected in a spiral fashion, and with channels for liquid cooling, pumped at high pressure. A magnitude of B = 45 T in air (equivalent to H = 36 MA/m) was achieved, but it required electrical power of 20 MW, at 67 kA of drive current.33)
Equation for the magnetic field inside the Bitter coil is different from that included below, because the current distribution in the copper disks cannot be assumed uniform, as is the case for a “thick” solenoid wound with a wire (because the current in each wire is the same due to serial connection, whereas in a Bitter coil higher current density is near the axis).34)
Interestingly, despite the progress with superconductors they still cannot be used directly for generation of such high fields, because the superconducting state collapses (becomes resistive) if the magnetic field exceeds the critical field.35) For this reason, hybrid approaches were utilised, combining the resistive Bitter solenoids and superconducting coils, allowing to reach up to 50 T of continuous field.36)
Even higher fields can be reached with pulsed (200 T) and explosive (1000 T) applications.
Calculator of H along axis of round "thick" solenoid
| | Stan Zurek, Calculator of H along axis of round “thick” solenoid, Encyclopedia Magnetica, https://www.e-magnetica.pl/doku.php/calculator/solenoid_thick, {accessed: 2025-09-27} |
| Magnetic field strength H of a circular “thick” solenoid along its axis | |
|---|---|
| Source: [1] Fausto Fiorillo, Measurement and Characterization of Magnetic Materials, Academic Press, 2005, ISBN 9780122572517 | |
| [1], eq. (4.27), p. 119 | |
| $$ H(t,x) = \frac{I(t) · N}{L·(D-d)}· \left( \left( \frac{L}{2}+x \right) ·\ln \frac{D+\sqrt{D^2+(L+2·x)^2}}{d+\sqrt{d^2+(L+2·x)^2}} + \left( \frac{L}{2}-x \right)·\ln \frac{D+\sqrt{D^2+(L-2·x)^2}}{d+\sqrt{d^2+(L-2·x)^2}} \right) $$ | (A/m) |
| where: $I(t)$ - current (A) at time $t$ (s), $N$ - total number of turns (unitless), $L$ - length of the solenoid (m), $d$ - inner diameter of the solenoid (m), $D$ - outer diameter of the solenoid (m), $x$ - location (m) from the centre of the solenoid (the centre is located at the point x = 0) | |
Notes: This equation is valid only for uniformly wound solenoid, with uniform current distribution in each turn. The instantaneous values of H are directly proportional to the instantaneous values of I. The value of B(μ0) is for vacuum.
| → → → Helpful page? Support us! → → → | PayPal | ← ← ← Help us with just $0.10 per month? Come on…  ← ← ← |
Rectangular solenoid

S. Zurek, E-Magnetica.pl, CC-BY-4.0
A solenoid can be also wound on a rectangular former. The calculation for the field is performed in a similar way as for a round solenoid - by using the field of the single rectangular loop of current (frame) and integrating over the length of the solenoid.37)
For a rectangular “thin” solenoid (negligible thickness of wire compared with the dimensions of the rectangular cross-section) the equation as shown below can be derived.38)
A solenoid with a square cross-section generates a field which is slightly lower than a round version with the same diameter as the length of the side of the square. However, a “flat” rectangle generates a field which is higher (i.e. closer to the ideal value of $H=N·I/L$), and provides uniformity over larger length along the axis, as illustrated in the graphs.39)
Rectangular solenoids are used for example in measurements of magnetic properties of soft magnetic materials. In the Epstein frame there are four rectangular coils, each placed in one side of a square. The strip samples are loaded such that they overlap. The “flat” rectangular solenoids (30 mm wide) provide uniform magnetising field over a large volume of the multi-strip sample.
Similarly, in the single sheet tester (SST) there is a single large coil, very “flat” (up to 500 mm long and 500 mm wide, around 5 mm thick) which uniformly magnetises the whole volume of the sample under test.40)
The equation for the rectangular solenoid can be also used for calculating the field outside of permanent magnets (see explanation in the next section). In literature of permanent magnets it is typically expressed with the fractions inverted and the terms subtracted in a reversed order, but otherwise returning identical numerical results.41)42)43)
The disadvantage of the different format is that the field cannot be calculated for the location of x = 0, whereas in the formula shown below such problem does not occur.
Calculator of H along axis of rectangular "thin" solenoid
| | Stan Zurek, Calculator of H along axis of rectangular “thin” solenoid, Encyclopedia Magnetica, https://www.e-magnetica.pl/doku.php/calculator/solenoid_rectangular, {accessed: 2025-09-27} |
| Magnetic field strength H of a rectangular “thin” solenoid along its axis | |
|---|---|
| Source: [1] Edward P. Furlani, Permanent magnet and electromechanical devices, Academic Press, London, 2021, ISBN 0122699513 | |
| [1], eq. (3.114), p. 135 (converted by S. Zurek to a current-fed solenoid and a different reference point along the axis) | |
| $$ H(t,x) = \frac{I(t) · N}{L·π}· \left( \text{atan} \frac{(2·x+L)·\sqrt{a^2+b^2+(2·x+L)^2}}{a·b} - \text{atan} \frac{(2·x-L)·\sqrt{a^2+b^2+(2·x-L)^2}}{a·b} \right) $$ | (A/m) |
| where: $I(t)$ - current (A) at time $t$ (s), $N$ - total number of turns (unitless), $L$ - length of the solenoid (m), $a \approx A$ and $b \approx B$ - length (m) of sides of the rectangular cross-section (inner and outer, as per the diagram), $x$ - location (m) from the centre of the solenoid (the centre is located at the point x = 0) | |
Notes: This equation is valid only for uniformly wound solenoid, with uniform current distribution in each turn. The instantaneous values of H are directly proportional to the instantaneous values of I. The value of B(μ0) is for vacuum. The same equation can be also used for rectangular permanent magnets if magnetisation is calculated to the units of amperes.
| → → → Helpful page? Support us! → → → | PayPal | ← ← ← Help us with just $0.10 per month? Come on…  ← ← ← |
Large solenoids

by A. Lampasi, F. Burini, G. Taddia, S. Tenconi, M. Matsukawa, K. Shimada, L. Novello, A. Jokinen, P. Zito, CC-BY-SA-4.0
Solenoid-like coils are used also in large devices such as tokamaks or particle accelerators.45)46)
Such solenoids can be several metres in size, and carry large currents, dissipating power in the range of MW (for short duty cycles).
In a tokamak, the central solenoid serves as a “backbone” of operation, inducing majority of the magnetic flux change required to initiate the plasma, generate the plasma current and sustain it during the burn.47)

by A. Romannikov and Fusion Research Centre Team, CC-BY-SA-4.0
Solenoidal field as a magnet

S. Zurek, E-Magnetica.pl, CC-BY-4.0
If the volume of permanent magnet can be assumed to be uniformly magnetised, then such magnet can be represented with an equivalent solenoid, effectively having the current flowing only on the surface, as illustrated.50)
The equivalency can be established because the magnetisation M can be related to the current density flowing in a “sheet” surrounding the volume of interest.51)
This equivalency is useful for performing numerical calculations involving magnets, because an equivalent electric current can be assigned to the given volume, and the numerical solution can be carried out as for any other energised coil.
For the same reason the analytical calculations can be performed with the same equations for permanent magnets and for solenoids, both round and rectangular.52)53)
If correctly expressed, the equations provide the magnetic field on the axis both outside, and inside of the magnets and the solenoids.

S. Zurek, E-Magnetica.pl, CC-BY-4.0
Solenoids actuators
Design of solenoid actuators follows the same rules as for any other electromagnetic actuators (e.g. relays), with the typical optimisation towards maximum force, over maximum distance, with minimum of energy required from the electrical supply.
Obviously, each design can be optimised to the specific application, as required.55)
Solenoid valves

by H-S. Lee, S-G. Park, M-P. Hong, H-J. Lee, Y-S. Kim, CC-BY-SA-4.0
The name “solenoid” is also widely used for electromagnetic actuators, especially for devices such as solenoid valves.57) The name “solenoid valve” is applied because the electromagnetic actuator is mechanically coupled to a hydraulic valve.
In such actuators, typically the main magnetising coil is used to provide the energy for generating useful linear force or torque. Typically, a plunger is attached to a spring which provides a returning force.
To improve the efficiency and the amount of generated force the device normally comprises a magnetic core to direct the magnetic field in to the main air gap. When the coil is energised the plunger is attracted, minimising the air gap and providing useful force or torque. After the coil is de-energised, the spring returns the plunger to the nominal position.
Permanent magnets can be also used in such actuators, in order to provide magnetic offset or facilitate operation in a “reversed” way (i.e. closing the valve with applied current, rather than opening it).58)

by H-S. Lee, S-G. Park, M-P. Hong, H-J. Lee, Y-S. Kim, CC-BY-SA-4.0
Other solenoid actuators
Solenoid-like coils are very widely used in magnetic measurements for generation of uniform and known magnetic field, and in electromagnetic devices, in which the distinction between a “solenoid” and a “winding” or “coil” no longer applies.
There are many specific technical reasons for which solenoids are used:
- maintaining a specific value of inductance in LC tuned circuits
- providing energy storage in magnetic field of chokes
- for sensing coils in induction sensors
- uniform magnetic field for measurements (magnetic, medical)
- and many more
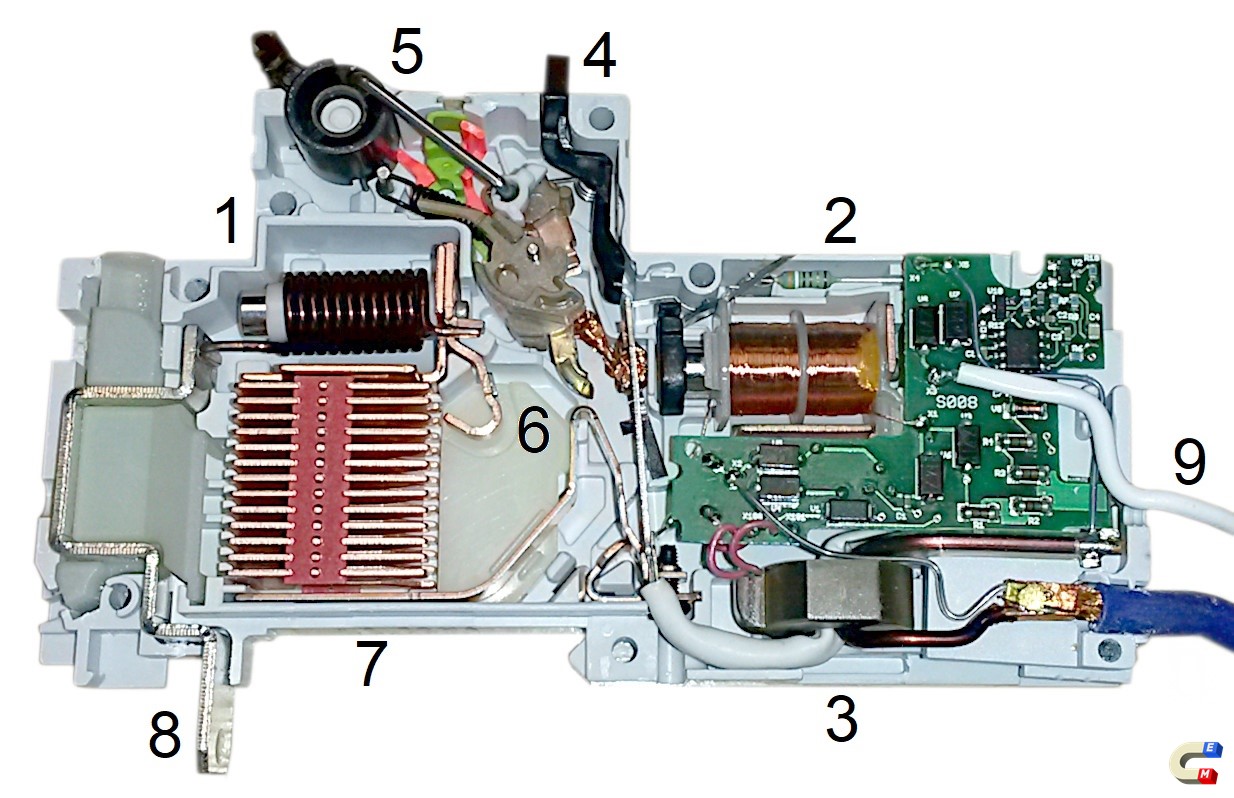
S. Zurek, E-Magnetica.pl, CC-BY-4.0
Examples of practical solenoids
 by B. Koprivica, Copyrights ©
by B. Koprivica, Copyrights ©
 by Daderot, Wikimedia Commons, CC0-1.0
by Daderot, Wikimedia Commons, CC0-1.0

S. Zurek, E-Magnetica.pl, CC-BY-4.0

S. Zurek, E-Magnetica.pl, CC-BY-4.0
S. Zurek, E-Magnetica.pl, CC-BY-4.0
















